I was planning for a teacher training program recently where I was tasked to include some community-building activities that could tie in with academic subjects. On my list were a few Cup activities – one being Line Um Up (from Cup It Up). It was an easy step to adapt this one into a mathematical challenge. After trying it out for the first time with this group of teachers, here’s what we have so far:
Solve For
Needs & Numbers:
Note: I’m suggesting Cups because I already have multiple sets of numbered cups ready for other Cup It Up activities. If you’re not a cupper yet with the resources ready, you can make number sets with index cards or small paper plates. I use the numbers 1 to 26 because Cup activities also use lettered sets, A to Z (26 cups here). So it's a good correlation.
On another note, the 'other colored' Solo Cups are usually around the stores during the Fall and Winter Holidays. (I'm also guessing you can get the colors online all-year-round?)
Set-Up: Check out the diagram below (the diagram below shows cups in play - before play, all cups will be at the Cup Stations). Prior to the activity, you’ll want to set up the Cup Stations you’ll need (one for every three to five players) – arrange the stations like the numbers on a traditional clock face. Then put your game spot (or something similar) in the center of the ‘clock.’ I like the spacing to be about 15 feet – from the game spot to the Cup Stations. Then, set one stack of 26 cups at each Cup Station. If the Cup Station surface is flat enough, stand up the cup stack open end up. If the stack won’t stand, simply lay it on its side in the cup station.
Solve For
Needs & Numbers:
- 26 numbered cups (see above) – numbered 1 to 26, for every group of three to five participants.
- One game spot (or something similar).
- One ‘Cup Station’ for each group: a taped rectangle on the floor, a hula-hoop, a small rope circle, or a table.
- One large sheet of paper (e.g., flip chart paper) and a marker.
- One or two index cards and a pen for each Cup Station.
- One timing device.
Note: I’m suggesting Cups because I already have multiple sets of numbered cups ready for other Cup It Up activities. If you’re not a cupper yet with the resources ready, you can make number sets with index cards or small paper plates. I use the numbers 1 to 26 because Cup activities also use lettered sets, A to Z (26 cups here). So it's a good correlation.
On another note, the 'other colored' Solo Cups are usually around the stores during the Fall and Winter Holidays. (I'm also guessing you can get the colors online all-year-round?)
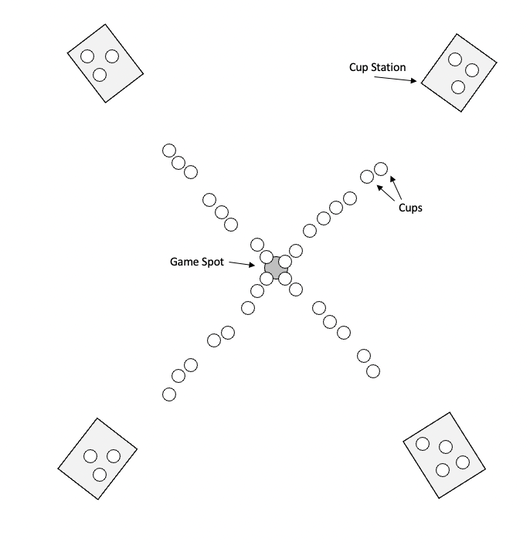
Set-Up: Check out the diagram below (the diagram below shows cups in play - before play, all cups will be at the Cup Stations). Prior to the activity, you’ll want to set up the Cup Stations you’ll need (one for every three to five players) – arrange the stations like the numbers on a traditional clock face. Then put your game spot (or something similar) in the center of the ‘clock.’ I like the spacing to be about 15 feet – from the game spot to the Cup Stations. Then, set one stack of 26 cups at each Cup Station. If the Cup Station surface is flat enough, stand up the cup stack open end up. If the stack won’t stand, simply lay it on its side in the cup station.
Process:
Why I Like This Activity?
Variations:
- Create groups of three to five participants. Have each group pick and stand by one of the Cup Stations. Tell them not to touch the cups until instructed to do so.
- Let everyone know they will be doing some math during the activity. (You might decide to discuss the physiological and psychological reactions to math at this time if it's noticeable.)
- Show all the groups the first set of four numbers listed on the large piece of paper (see header picture for an example).
- The objective, for each Cup Station team, is to solve for each number on the list using the numbered cups at their station. A formula – using more than one cup – must be created to solve for each number.
- Any mathematical symbols can be used along with the numbers to reach the sum needed – the numbers on the list. (The symbols are invisible, or assumed.)
- Formulas must be made in the order of the numbers on the list. So, (using the second list of numbers in the header picture, for example), solve for 15 first. The first cup of the formula for 15 must be placed on the game spot, and then the next cup or cups follow in line from the game spot towards the team's Cup Station (see the diagram above).
- Write down your formula for each number on an index card so the formulas can be verified at the end of each round.
- After the first formula of cups is placed down, the group can work on solving for the next number. When this next formula is ready it can be placed down in the line – leaving a noticeable space between formulas.
- When all teams are ready – after answering questions – you can call "GO!" and start the timer. Team members can then touch their cups.
- Only the cups of a formula can be out in the playing area (in line). All other cups must stay on/in the Cup Station during the solving phase of the formulas.
- Once all teams have placed down a formula for each number on the list, the time stops. This is an important distinction – the time does not stop until all teams have 'Solved For' all numbers in the Round.
- Someone (or more than one) from each team will then verbally share the formulas for each number on the list – use the index card if needed. If a formula is incorrect ask the group what they will need to do to fix it – then have them fix it. (There is no penalty/consequence.)
- Once all the formulas have been verified (and fixed if needed), share the overall time with the group. (The first time recorded with three teams in play was 2 min., 37 seconds, using the first list of numbers in the header picture above.)
- This 'overall time' (as I'm suggesting for now), is the baseline for the remaining Rounds. (Same expectation for more work. Fair?)
- Round one is four numbers. Round two is five numbers. Round three is six numbers, and round four is seven numbers. Each Round starts the same way as Round one. NOTE: So far there is not an 'ideal' set of four lists of numbers – will a particular list of seven numbers be impossible to solve for? (See the Variation below to provide more resources to work with.)
Why I Like This Activity?
- When we use an 'all group' time, there is a better chance for collaboration to take place in order to meet the time expectation.
- Using math 'problems' to solve can bring up some good discussions about skills, abilities, and mindsets, as well as roles and responsibilities. If someone is 'not good' at math, what role do they play during each Round?
- Using the overall baseline time as the benchmark for each Round will, most likely, force collaboration in order to meet the expectation. And what compromises will be made (e.g., skill acquisitions) for the sake of the expectation? I'm thinking, because we have to meet a 'standard' the participants who excel at math will do the work – others will not get the practice or the encouragement to practice.
- I'm curious to find out if some groups will not care about the expectations and focus on the 'process' of including everyone along the way – everyone feels they contributed to the results.
Variations:
- Use index cards for the number list they will solve for during each Round (instead of writing the numbers on one sheet of paper). Give each group the same list of numbers, but don't inform them they are the same – if the groups share this information, it's okay. And maybe will lead to some collaboration?
- What if you put all the 'math-smart' participants in one (or two) teams? How long will it take, without collaborating, to solve for all the numbers? (Q: What are some of the advantages of diversity?)
- Allow teams to use the last number in one formula to be the first number in the next formula. This 'resource' is useful for longer lists of numbers (e.g., 6 to 8 numbers).
If you give this one a try. Let us know what else can be done. Leave a Comment.
All the best,
Chris Cavert, Ed.D.
All the best,
Chris Cavert, Ed.D.
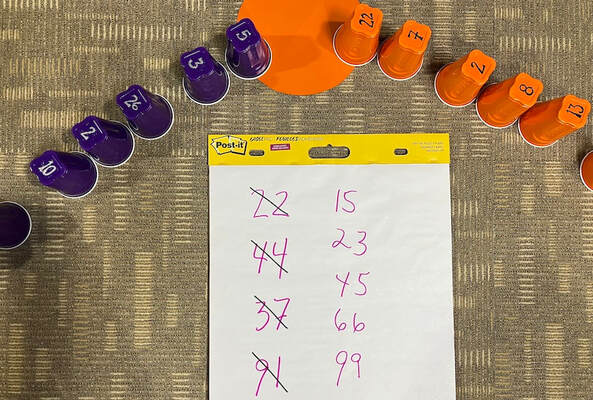
RSS Feed